(To Japanese)

A kind introduction to self-inducing systems:

Matter can sometimes form ordered structures at low temperatures when certain conditions are met. The oldest known example of such order is the crystal structure, but in some cases, there exists a quasicrystal, which does not have periodicity yet exhibits large scale repetition. My interest lies in the mathematics that arises at the boundary between the periodic and non-periodic structures. Even in pure mathematics, phenomena can sometimes be observed at the boundary between non-periodicity and periodicity, often leading to astonishing consequences. As an example, in the Markoff-Lagrange spectrum in the theory of Diophantine approximations, as well as in the recently discovered aperiodic monotile, a non-periodic structure called a Sturmian sequence emerges. Keywords for describing such structures are quasi-periodic structure and self-similarity. I am particularly interested in the field related to quasi-periodic tiling. Looking at some figures.

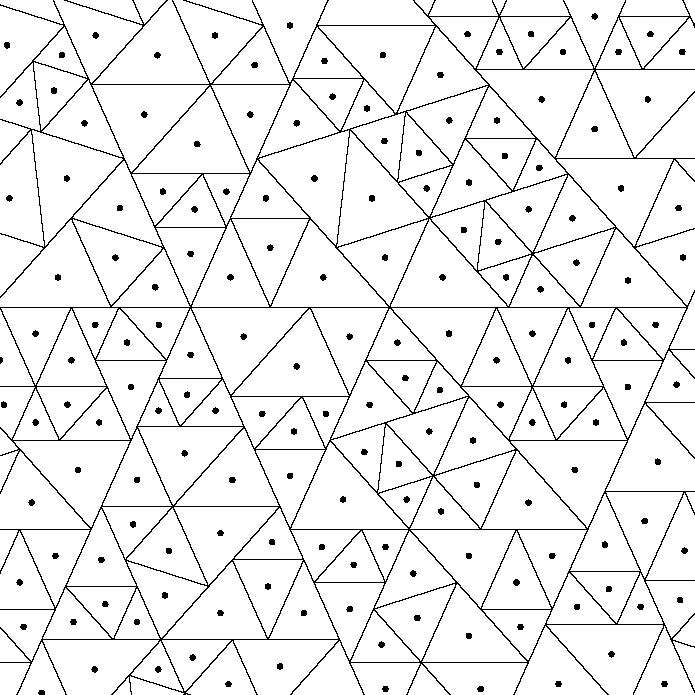
Left: Badly approximable triangles Right: Dragon Curve